|
Strange Attractors_2018
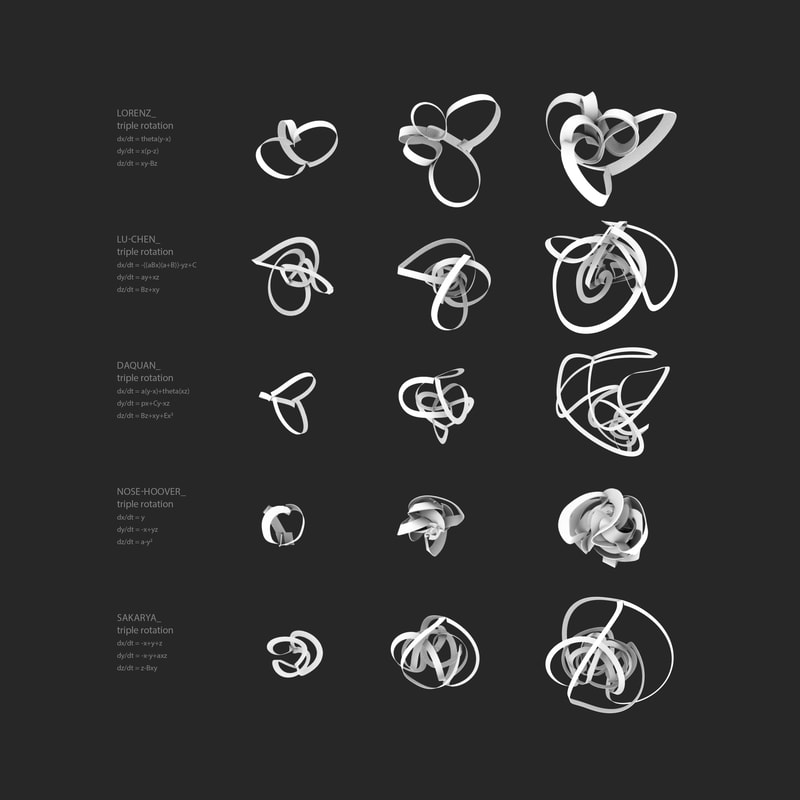
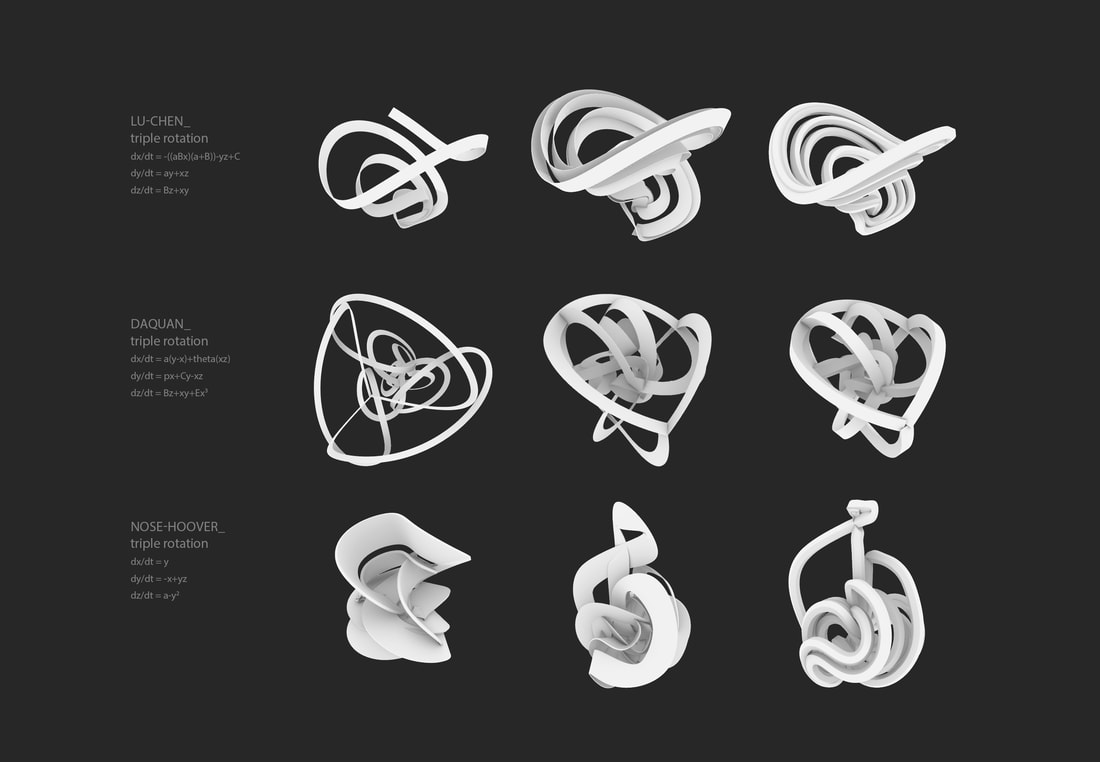
Free exploration / Matt Walker / Sumer Singh An exploration of utilizing the foundations of chaos theory to create and justify form. A patterned form arising on the basis of mathematical appropriations of a chaotic system is also known as a "Strange Attractor", which is fundamentally a system that is highly sensitive to its initial conditions. Ultimately, it is representative of chaotic systems with discrete, underlying parameters that essentially 'program" the final forms. Initial explorations revolved around point limits; the number of points that the chaotic system could produce in order to generate extents of its shape. Ranging from 50 o 300 points within the strange attractor's chaotic system, flat geometries were first generated using triple rotation to investigate inherently asymmetric configurations. These explorations were pushed to the absolute extents of Grasshopper's capacity to create several chaotic systems: Lorenz, Lu-chen, Daguan, Nose-hoover, Sakarya. The outcome of this research is the rationalization of the strange attractor's formal output as a light fixture using computationally driven segmentation strategies. In a fully automated and parametric process, complex chaotic geometry can be segmented and modified to create channels for lighting, segmented into 3D-printable parts, and fitted with smart joinery systems allowing for easy assembly of complex forms without additional formwork. 
physical outcomes
chaotic iterations
selected iterations
fabrication strategy
|